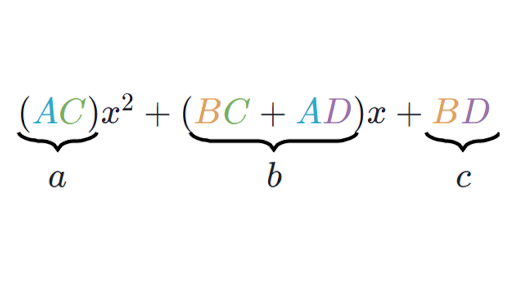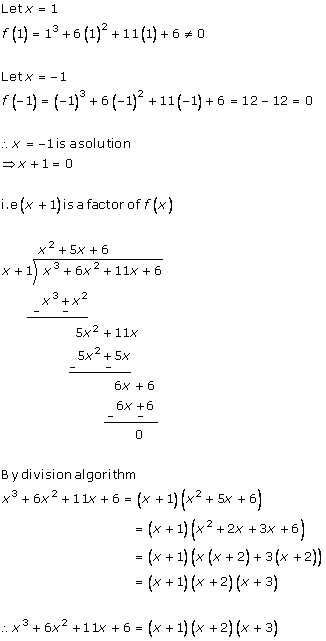How To Factor Polynomials X Method

The correct factoring of this polynomial is x2 20x 100 left x 10 right2 to be honest it might have been easier to just use the general process for factoring quadratic polynomials in this case rather than checking that it was one of the special forms but we did need to see one of them worked.

How to write the essay. 1 3 41 2 71 10 0. For all polynomials first factor out the greatest common factor gcf. A common method for multiplying the two binomials together is called foil and the factoring of the resulting trinomial is often referred to as unfoil.

6ax 9ay 2x 4y here from the first two terms take 3a as common and form 3rd and 4th term take 2 as common. An expression of the form ax n bx n 1 cx n 2 kx l where each variable has a constant accompanying it as its coefficient is called a polynomial of degree n in variable x. Find the gcf of the two new terms.

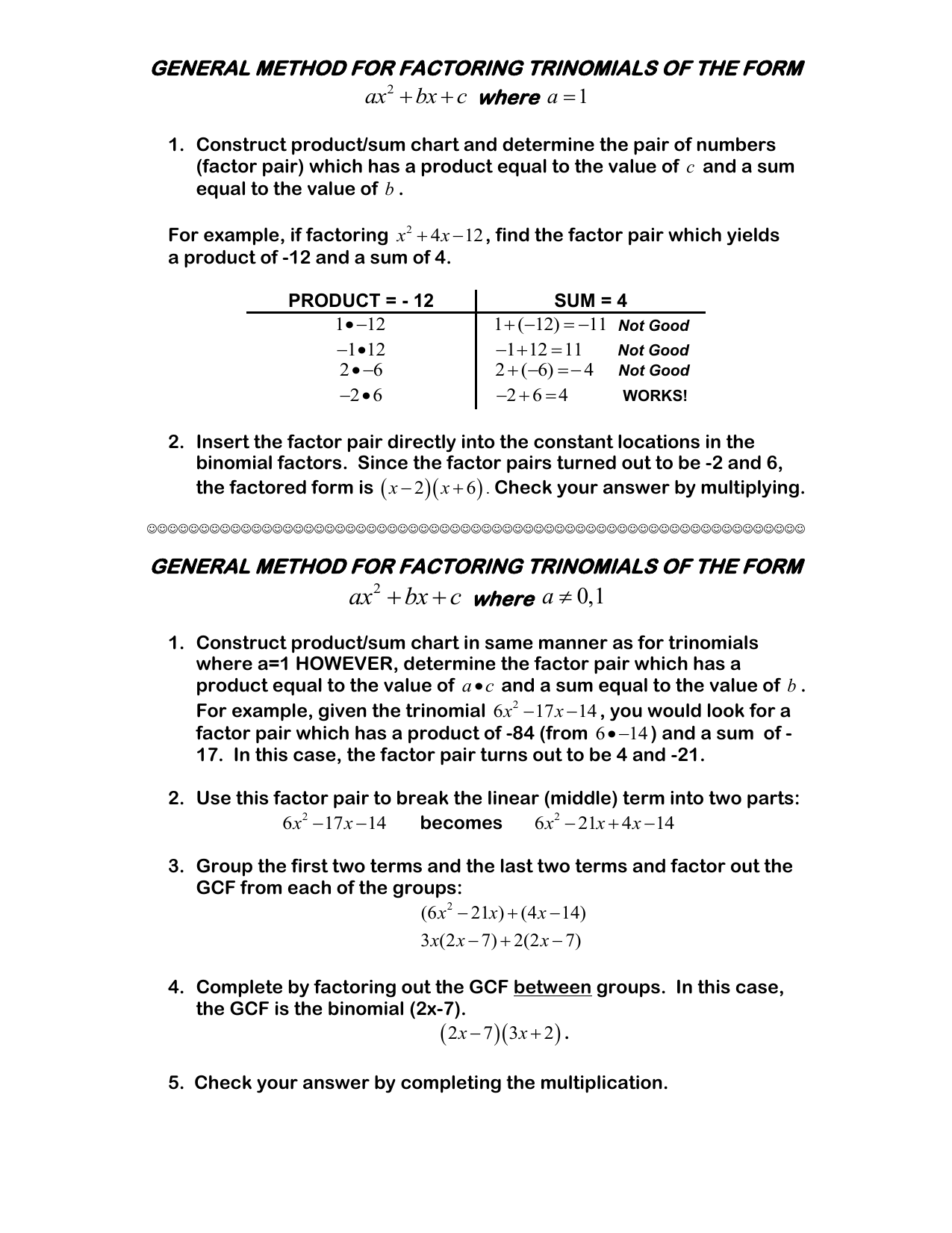
X 2 8x 15 x 3 x 5. 1 4 7 10 0. For a binomial check to see if it is any of the following.

X 2 and x 4 are the two roots of the given polynomial of degree 4. Thats a gcf because it appears in both terms if you factor using this method the last step should always look. The polynomial is now written as xx 2 5x 2.

Find one factor that causes the polynomial to equal to zero. Suppose we want to factor 2x 2 5x 3. You still need to know the factors of a and c but the box method gives you a more systematic process for determining which factors and terms to choose.

This method is used when there is no factor common to all the terms of a polynomial but there will be factors common to some of the terms. Factoring polynomials is the reverse procedure of multiplication of factors of polynomials. For the second case there isnt really a much better method than to just multiply it all out.

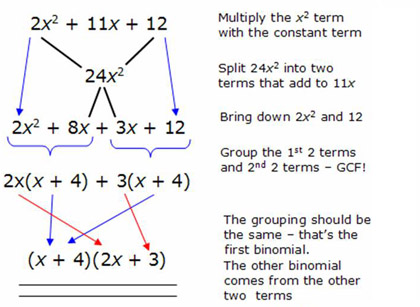
1 first we draw an x shape our diamond. The diamond method provides another systematic way to factor second degree polynomials of the form ax 2 bx c that are hard that is those second degree polynomials that have a value for a other than 1. This method is best illustrated using an example.

Start by using your first factor 1. Do you see the x 2 in both terms. To find other roots we have to factorize the quadratic equation x 2 8x 15.

If you factor out the 5 you have 5x 2. Thus a polynomial is an expression in which a combination of a constant and a variable is separated by an. X 2 y 2 x y x y difference of cubes.

We want to determine which factor makes the polynomial equal zero when we substitute the factor for each x in the equation. X 3 y 3 x y x 2 xy y 2 sum of cubes. X2 a1 x a0x2 b1 x b0 x4 c3 x3 c2 x2 c1 x c0 where weve introduced for notational cleanliness i had the computer multiply this out for me because its there.

X 3 y 3 x y x 2 xy y 2 for a trinomial check to see whether it is either of the following forms. Substitute 1 for each x in the equation.